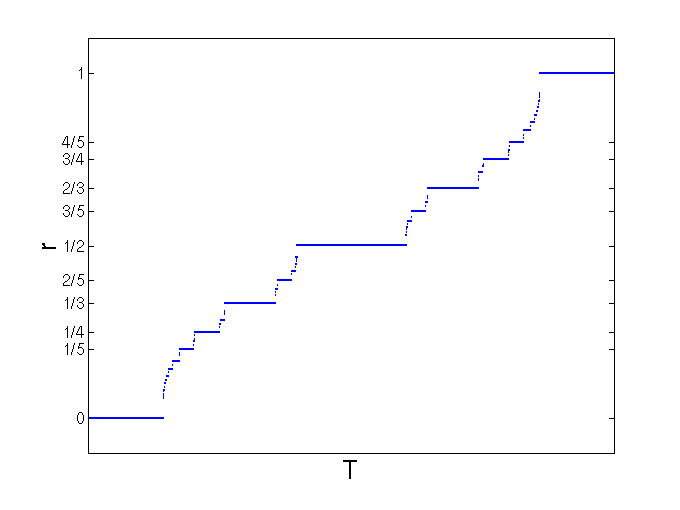
A complete devil's staircase is a graph that is flat every where, except to an infinite (but countable) number of point. It contains plateaus for all rational numbers between 0 and 1. The length of these plateaus are generally wider as the denominator of the rational number (in its reduced form) is smaller.

The diabolic scheme here is that even though the length and height of the staircase is finite, there is an infinite number of steps, so whoever is tempted to climb this staircase will spend eternity to reach its end.

Last updated on June 13, 2003